Mounted N-BK7 Bi-Convex Lenses (Uncoated)

- Positive Focal Length for Use at Finite Conjugates
- Uncoated Wavelength Range: 350 - 2000 nm
- SM05-Threaded or SM1-Threaded Mounts
LB1869-ML
(Ø1")
LB1187-ML
(Ø1/2")

Please Wait
| N-BK7 Bi-Convex Spherical Singlets | |
|---|---|
| Lens Shape | Convex/Convex |
| Material | N-BK7 (Grade A) |
| Wavelength Rangea | 350 nm - 2.0 μm (Uncoated) |
| Design Wavelength | 587.6 nm |
| Index of Refraction | 1.517 (@ 587.6 nm) |
| Surface Quality | 40-20 Scratch-Dig |
| Surface Irregularityb | λ/4 |
| Spherical Surface Powerb | 3λ/2 |
| Abbe Number | Vd = 64.17 |
| Centration | ≤3 arcmin |
| Clear Aperture | Ø1/2" lenses: >Ø11.04 mm Ø1" lenses: >Ø22.86 mm |
| Diameter Tolerance | +0.00 mm / -0.10 mm |
| Focal Length Tolerance | ±1% |
| Click on the red Document icon next to the item numbers below to access the Zemax file download. Our entire Zemax Catalog is also available. |
Features
- Material: N-BK7
- Wavelength Range: 350 - 2000 nm (Uncoated)
- Available Sizes: Ø1/2" and Ø1"
- Focal Lengths Available from 15.0 mm to 1.0 m
- Well Suited for Many Finite Imaging Applications
- Offers Excellent Transmittance Throughout the Visible and Near Infrared
- Mounted in SM-Compatible Lens Cells
Thorlabs' Mounted Uncoated N-BK7 Bi-Convex Lenses are popular for many finite imaging applications. This type of lens is best suited for use in situations where the object and image are on opposite sides of the lens and the ratio of the image and object distances (conjugate ratio) is between 0.2 and 5.
N-BK7 is probably the most common optical glass used for high-quality optical components. It is typically chosen whenever the additional benefits of UV fused silica (i.e., good transmission further into the UV and a lower coefficient of thermal expansion) are not necessary. These Ø1/2" and Ø1" lenses are mounted in SM05- (0.535"-40) and SM1- (1.035"-40) compatible mounts, respectively.
Although this page features our uncoated bi-convex lenses, these lenses are also available with one of four antireflection coatings (-A, -AB, -B, or -C) deposited on both sides to reduce the amount of light reflected from each surface. Links to each of these pages can be found in the table to the right. Please see the Graphs tab for coating details.
Lens kits are available. Please click here for information.
| Bi-Convex Lens Selection Guide | |
|---|---|
| N-BK7 | |
| Mounted N-BK7 |
Uncoated
-A (350 - 700 nm) |
| UV Fused Silica | |
| CaF2 | |
| ZnSe | -E3 (7 - 12 µm) |
Custom coatings are also available. Please contact Tech Sales for a quote.
| Quick Links to Other Spherical Singlets | ||||||
|---|---|---|---|---|---|---|
| Plano-Convex | Bi-Convex | Best Form | Plano-Concave | Bi-Concave | Positive Meniscus | Negative Meniscus |
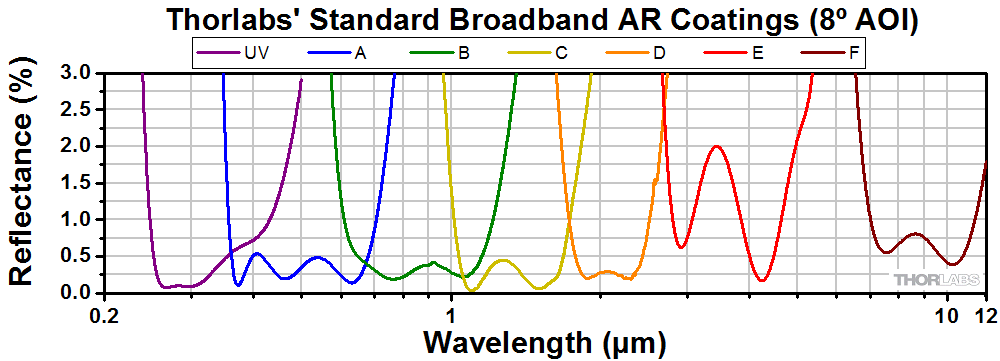
Below is the transmission curve for N-BK7, a RoHS-compliant form of BK7. Total Transmission is shown for a 10 mm thick, uncoated sample and includes surface reflections. Each N-BK7 bi-convex lens can be ordered uncoated or with one of the following broadband AR coatings: 350 - 700 nm (designated as -A), 400 - 1100 nm (designated as -AB), 650 - 1050 nm (designated as -B), or 1050 - 1700 nm (designated as -C).
Our high-performance multilayer AR coatings have an average reflectance of less than 0.5% (per surface) across the specified wavelength ranges and provide good performance for angles of incidence (AOI) between 0° and 30° (0.5 NA). For optics intended to be used at large incident angles, consider using a custom coating optimized at a 45° angle of incidence; this custom coating is effective from 25° to 52°. The plot shown below indicates the performance of the standard coatings in this family as a function of wavelength. Broadband coatings have a typical absorption of 0.25%, which is not shown in the reflectance plots.

Click to Enlarge
Click Here for Raw Data
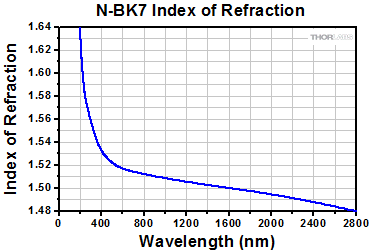
Click here for detailed substrate data.
In the thick lens equation, use the index of refraction for N-BK7 at the wavelength of interest to approximate the wavelength-dependent focal length of any of the bi-convex lenses.
The focal length of a thick spherical lens can be calculated using the thick lens equation below. In this expression, nl is the index of refraction of the lens, R1 and R2 are the radii of curvature for surfaces 1 and 2, respectively, and d is the center thickness of the lens.
![]()
For bi-convex lenses, where the front and back radii of curvature are equal in magnitude and opposite in sign, R1 = -R2 = R, we have
![]()
The focal length of the lens calculated using the simplified thick lens equation directly above is the distance between the second (back) principle plane (H") and the position at which a collimated beam incident on the curved surface of the bi-convex is focused. The same analysis can be performed using a point source and the first (front) principle plane (H'). The principle plane positions of a thick lens can be calculated with the following equations:
![]() and
and ![]()
Again, for the same bi-convex lens, R1 = -R2 = R, we can solve for H' and H' :
![]()
| Posted Comments: | |
| No Comments Posted |


 Products Home
Products Home








 N-BK7, Mounted, Uncoated
N-BK7, Mounted, Uncoated